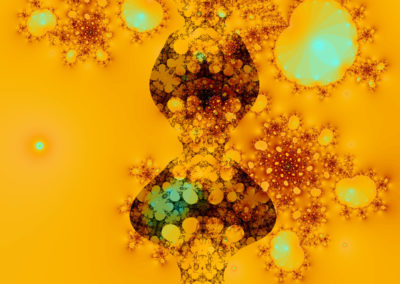
Fractal 078 C – Hell’s Gate

Part of BEYOND series – 04 2022

cathedral . fear . frontier . gate . heat . hell . lava . magma

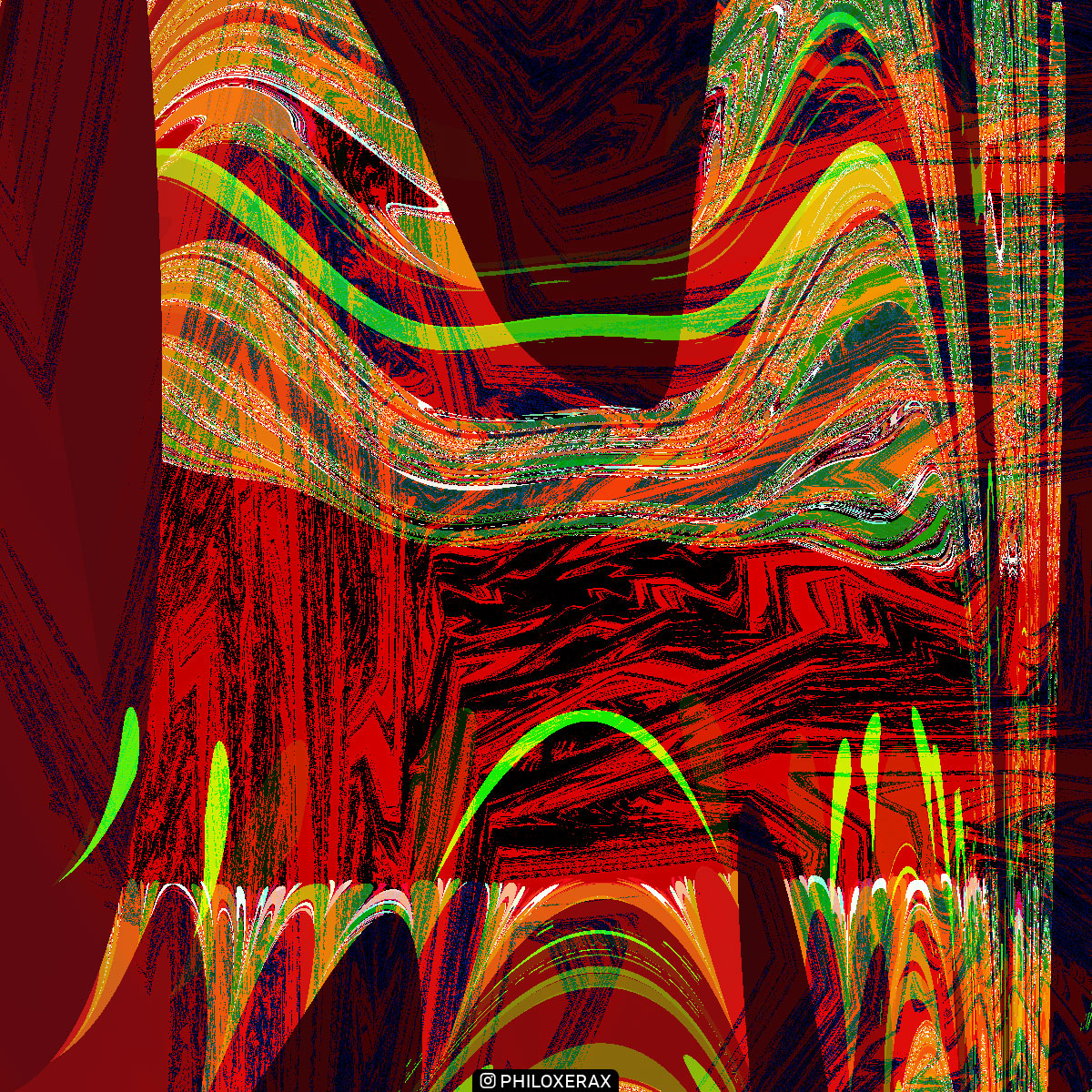
← Fractal 075 D - Light vs Darkness Fractal 091 A - Guard of the Underworld →

Fractal 078 C – zoom level 1

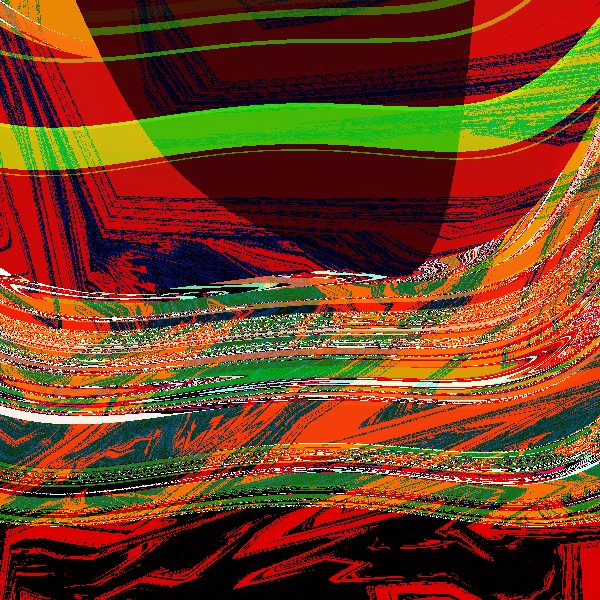
Fractal 078 C – zoom level 2

Previous versions

Fractal 078 B – Under World | Part of BEYOND series | 04.2023

Fractal 078 A – Under World | Part of BEYOND series | 03.2023

Data

This digital works is created exclusively from fractals, with 0% AI generated. It can therefore be converted into a set of functions and parameters that can be the basis for the training of an AI.

The mathematical formulas and parameter combinations corresponding to each fractal are presented below. In each case, the numerical characters have been replaced by ■ to prevent unauthorized reproduction. If you are interested in using the full data set to train an AI, please contact Philippe.

Fractal_078_C {
fractal:
  title="Fractal_078_C" width=████ height=████ layers=█
  credits="Philoxerax;6/9/2023"
layer:
  caption="Layer 3" opacity=███ mergemode=lighten
mapping:
  center=-█.█████████████/█.████████████ magn=███.█████
  angle=-███.████
formula:
  maxiter=███ entry="mt-990807a-m" p_e1=█.█ p_e2=█.█
  p_bailout=████.█ f_fn1=flip f_fn2=ident
inside:
  transfer=none
outside:
  transfer=linear
gradient:
  smooth=yes rotation=-█ index=███ color=█ index=███ color=█████
  index=███ color=█ index=███ color=█ index=███ color=█ index=███
  color=█ index=███ color=█ index=███ color=█
opacity:
  smooth=no index=█ opacity=███
layer:
  caption="Background" opacity=███ mergemode=overlay
mapping:
  center=-█.█████████████/█.█████████████ magn=███.█████
  angle=-██.████
formula:
  maxiter=███ 
  entry="mixed-up-multiplication-mandelbrot" p_power=█ p_bailout=████
  p_shape_type=square p_q1_type=out p_q2_type=out p_q3_type=out
  p_q4_type=out p_freq=█ p_amp=█.█ p_nteeth=█ p_rsmall=█.█
  p_inrad=█.██
inside:
  transfer=none
outside:
  transfer=linear
gradient:
  smooth=yes rotation=-██ index=██ color=███████ index=███
  color=██████ index=███ color=███████ index=-██ color=███████
opacity:
  smooth=no index=█ opacity=███
layer:
  caption="Layer 2" opacity=██ mergemode=red
mapping:
  center=-█.█████████████/█.█████████████ magn=███.█████
  angle=-███.████
formula:
  maxiter=███ entry="mt-990807a-m" p_e1=█.█ p_e2=█.█
  p_bailout=████.█ f_fn1=flip f_fn2=ident
inside:
  transfer=none solid=██████████
outside:
  transfer=linear
gradient:
  smooth=yes rotation=███ index=███ color=███████ index=███
  color=███████ index=███ color=███████ index=███ color=████████
  index=███ color=████████ index=███ color=███████ index=███
  color=████████ index=███ color=████████ index=███ color=█████
  index=███ color=███████ index=███ color=████████
opacity:
  smooth=no index=█ opacity=███
layer:
  caption="Background" opacity=███
mapping:
  center=-█.█████████████/█.█████████████ magn=███.█████
  angle=-███.████
formula:
  maxiter=███ entry="mt-990807a-m" p_e1=█.█ p_e2=█.█
  p_bailout=████.█ f_fn1=flip f_fn2=ident
inside:
  transfer=none
outside:
  transfer=linear
gradient:
  smooth=yes rotation=███ index=███ color=███████ index=███
  color=███████ index=███ color=███████ index=███ color=███████
  index=███ color=██████ index=███ color=███████ index=███
  color=███████ index=███ color=████████ index=███ color=████████
  index=███ color=███████ index=███ color=████████
opacity:
  smooth=no index=█ opacity=███
}