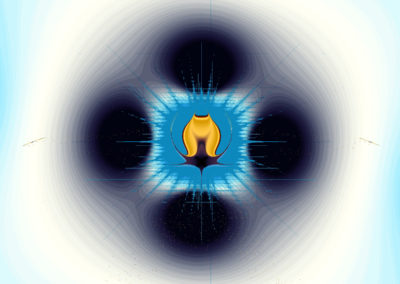
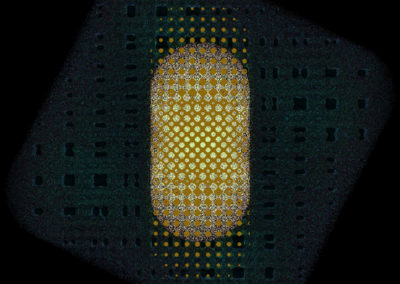
Fractal 098 E – Orgasm

Part of DIALOG series – 04 2023

alchemy . bliss . color . delaunay . flesh . orgasm
Fractal 098 E | Orgasm
Fractal 098 E zoom1

Fractal 098 E – zoom level 1

Fractal 098 E zoom2

Fractal 098 E – zoom level 2

Data

This digital works is created exclusively from fractals, with 0% AI generated. It can therefore be converted into a set of functions and parameters that can be the basis for the training of an AI.

The mathematical formulas and parameter combinations corresponding to each fractal are presented below. In each case, the numerical characters have been replaced by â–  to prevent unauthorized reproduction. If you are interested in using the full data set to train an AI, please contact Philippe.

Fractal 098 E - Orgasm - Part of DIALOG series - 04.2023

Fractal 098 E | Orgasm

Fractal_â– â– â– _E {
fractal:
  title="Fractal_â– â– â– _E" width=â– â– â– â–  height=â– â– â– â–  layers=â– 
  credits="Philoxerax;â– /â– â– /â– â– â– â– " antialiasing=yes
layer:
  caption="Layer â– " opacity=â– â– â–  transparent=yes
mapping:
  center=â– .â– â– â– â– â– â– â– â– â– â– â– â– â– â– â– â– â– â– â– /â– .â– â– â– â– â– â– â– â– â– â– â– â– â– â– â– â– â– â– â–  magn=â– .â– â– â– â– â– â– â– Eâ– 
  angle=â– â– â– .â– â– â– â– 
formula:
  maxiter=â– â– â– â–  percheck=off filename="dmj.ufm"
  entry="dmj-PhoenixDNovaJulia" p_seed=â– /â–  p_powerâ– =â– /â–  p_powerâ– =â– /â– 
  p_coeffâ– =â– /â–  p_coeffâ– =-â– /â–  p_induct=-â– .â– /â–  p_bailout=â– .â– â– â– â– â– 
  p_relax=â– /â– 
inside:
  transfer=none solid=â– â– â– â– â– â– â– â– â– â– 
outside:
  transfer=linear
gradient:
  smooth=yes rotation=â– â–  index=â– â–  color=â–  index=â– â– â–  color=â–  index=â– â– â– 
  color=â–  index=â– â– â–  color=â–  index=â– â– â–  color=â– 
opacity:
  smooth=no index=â–  opacity=â– 
layer:
  caption="Layer â– " opacity=â– â– â–  mergemode=softlight
mapping:
  center=â– .â– â– â– â– â– â– â– â– â– â– â– â– â– â– â– â– /â– .â– â– â– â– â– â– â– â– â– â– â– â– â– â– â– â–  magn=â– â– â– â– â– â– â– .â– 
  angle=â– â– â– .â– â– â– â– 
formula:
  maxiter=â– â– â– â–  percheck=off filename="dmj.ufm"
  entry="dmj-PhoenixDNovaJulia" p_seed=â– /â–  p_powerâ– =â– /â–  p_powerâ– =â– /â– 
  p_coeffâ– =â– /â–  p_coeffâ– =-â– /â–  p_induct=-â– .â– /â–  p_bailout=â– .â– â– â– â– â– 
  p_relax=â– /â– 
inside:
  transfer=none solid=â– â– â– â– â– â– â– â– â– â– 
outside:
  transfer=linear
gradient:
  smooth=yes rotation=â– â–  index=â– â– â–  color=â– â– â– â– â– â– â–  index=â– â– â– 
  color=â– â– â– â– â– â– â–  index=â– â– â–  color=â– â– â– â– â– â– â– â–  index=â– â– â–  color=â– â– â– â– â– â– â– â– 
  index=â– â– â–  color=â– â– â– â– â– â– â– â–  index=â– â– â–  color=â–  index=â– â– â–  color=â– â– â– â– â– â– â– â– 
  index=â– â– â–  color=â– â– â– â– â– â– â–  index=â– â– â–  color=â– â– â– â– â– â– â–  index=â– â– â– 
  color=â– â– â– â– â– â– â– â–  index=â– â–  color=â– â– â– â– â– â– â– â–  index=â– â– â–  color=â– â– â– â– â– â– â– â– 
  index=â– â– â–  color=â– â– â– â– â– â– â– â–  index=â– â– â–  color=â– â– â– â– â– â–  index=â– â– â– 
  color=â– â– â– â– â– â– â–  index=â– â– â–  color=â– â– â– â– â– â– â– â–  index=â– â– â–  color=â– â– â– â– â– â– â– â– 
  index=â– â– â–  color=â– â– â– â– â– â– â– â–  index=â– â– â–  color=â– â– â– â– â–  index=â– â– â– 
  color=â– â– â– â– â– â– â–  index=â– â– â–  color=â– â– â– â– â– â– â– â–  index=â– â– â–  color=â– â– â– â– â– â– â– â– 
  index=â– â– â–  color=â– â– â– â– â–  index=â– â– â–  color=â– â– â– â– â– â– â– â–  index=â– â– â– 
  color=â– â– â– â– â– â– â–  index=â– â– â–  color=â– â– â– â– â– â– â–  index=â– â– â–  color=â– â– â– â– â– â– â– 
  index=â– â– â–  color=â– â– â– â– â– â– â–  index=â– â– â–  color=â– â– â– â– â– â– â–  index=â– â– â– 
  color=â– â– â– â– â– â– â– â– 
opacity:
  smooth=no index=â–  opacity=â– â– â– 
layer:
  caption="Layer â– " opacity=â– â–  mergemode=overlay
mapping:
  center=â– .â– â– â– â– â– â– â– â– â– â– â– â– â– â– â– â– â– â– /â– .â– â– â– â– â– â– â– â– â– â– â– â– â– â– â– â– â– â–  magn=â– .â– â– â– â– â– â– â– Eâ– 
  angle=â– â– â– .â– â– â– â– 
formula:
  maxiter=â– â– â– â–  percheck=off filename="dmj.ufm"
  entry="dmj-PhoenixDNovaJulia" p_seed=â– /â–  p_powerâ– =â– /â–  p_powerâ– =â– /â– 
  p_coeffâ– =â– /â–  p_coeffâ– =-â– /â–  p_induct=-â– .â– /â–  p_bailout=â– .â– â– â– â– â– 
  p_relax=â– /â– 
inside:
  transfer=none solid=â– â– â– â– â– â– â– â– â– â– 
outside:
  transfer=linear
gradient:
  smooth=yes rotation=â– â–  index=â– â–  color=â– â– â– â– â– â– â–  index=â– â– â– 
  color=â– â– â– â– â– â– â– â–  index=â– â– â–  color=â– â– â– â– â– â– â– â–  index=â– â– â–  color=â– â– â– â– â– â– â– â– 
  index=â– â– â–  color=â– â– â– â– â– â– â– â– 
opacity:
  smooth=no index=â–  opacity=â– â– â– 
layer:
  caption="Layer â– " opacity=â– â– â–  mergemode=luminance
mapping:
  center=â– .â– â– â– â– â– â– â– â– â– â– â– â– â– â– â– â– â– â– â– â– /â– .â– â– â– â– â– â– â– â– â– â– â– â– â– â– â– â– â– â– â–  magn=â– .â– â– â– â– â– â– â– Eâ– 
  angle=â– â– â– .â– â– â– â– 
formula:
  maxiter=â– â– â– â–  percheck=off filename="dmj.ufm"
  entry="dmj-PhoenixDNovaJulia" p_seed=â– /â–  p_powerâ– =â– /â–  p_powerâ– =â– /â– 
  p_coeffâ– =â– /â–  p_coeffâ– =-â– /â–  p_induct=-â– .â– /â–  p_bailout=â– .â– â– â– â– â– 
  p_relax=â– /â– 
inside:
  transfer=none
outside:
  transfer=linear
gradient:
  smooth=yes rotation=â– â–  index=â– â–  color=â– â– â–  index=â– â– â–  color=â– â– â– â– â– â– â– 
  index=â– â– â–  color=â– â– â– â– â– â– â– â–  index=â– â– â–  color=â– â– â– â– â– â– â– â–  index=â– â– â– 
  color=â– â– â– 
opacity:
  smooth=no index=â–  opacity=â– â– â– 
layer:
  caption="Layer â– " opacity=â– â–  mergemode=blue
mapping:
  center=â– .â– â– â– â– â– â– â– â– â– â– â– â– â– â– â– /â– .â– â– â– â– â– â– â– â– â– â– â– â– â– â– â– â–  magn=â– â– â– â– â– â– â– 
  angle=â– â– â– .â– â– â– â– 
formula:
  maxiter=â– â– â– â–  percheck=off filename="dmj.ufm"
  entry="dmj-PhoenixDNovaJulia" p_seed=â– /â–  p_powerâ– =â– /â–  p_powerâ– =â– /â– 
  p_coeffâ– =â– /â–  p_coeffâ– =-â– /â–  p_induct=-â– .â– /â–  p_bailout=â– .â– â– â– â– â– 
  p_relax=â– /â– 
inside:
  transfer=none
outside:
  transfer=linear
gradient:
  smooth=yes rotation=â–  index=â–  color=â– â– â– â– â–  index=â– â– â–  color=â– â– â– â– â– â– â– â– 
  index=â– â– â–  color=â– â– â– â– â– â– â– â–  index=â– â– â–  color=â– â– â– â– â– â– â–  index=â– â– â– 
  color=â– â– â– â– â– â– â–  index=â– â– â–  color=â– â– â– â– â– â– â–  index=â– â– â–  color=â– â– â– 
opacity:
  smooth=no index=â–  opacity=â– â– â– 
layer:
  caption="Layer â– " opacity=â– â– â– 
mapping:
  center=â– .â– â– â– â– â– â– â– â– â– â– â– â– â– â– â– â– â– â– â– â– /â– .â– â– â– â– â– â– â– â– â– â– â– â– â– â– â– â– â– â– â– â–  magn=â– .â– â– â– â– â– Eâ– 
  angle=â– â– â– .â– â– â– â– 
formula:
  maxiter=â– â– â– â–  percheck=off filename="dmj.ufm"
  entry="dmj-PhoenixDNovaJulia" p_seed=â– /â–  p_powerâ– =â– /â–  p_powerâ– =â– /â– 
  p_coeffâ– =â– /â–  p_coeffâ– =-â– /â–  p_induct=-â– .â– /â–  p_bailout=â– .â– â– â– â– â– 
  p_relax=â– /â– 
inside:
  transfer=none solid=â– â– â– â– â– â– â– â– â– â– 
outside:
  transfer=linear
gradient:
  smooth=yes rotation=â– â–  index=â– â–  color=â– â– â– â– â–  index=â– â–  color=â– â– â– â– â– â– 
  index=â– â– â–  color=â– â– â– â– â– â– â– â–  index=â– â– â–  color=â– â– â– â– â–  index=â– â– â– 
  color=â– â– â– â– â– â– â– â–  index=â– â– â–  color=â– â– â– â– â– â– â– 
opacity:
  smooth=no index=â–  opacity=â– â– â– 
}

dmj-PhoenixDNovaJulia {
;
; This is the DoubleNova fractal (Julia form), a
; modified Newtonian-style fractal.
;
; This variant includes an inductive component similar
; to the Phoenix fractal.
;
init:
  complex zold = (â– ,â– )
  complex y = (â– ,â– )
  
  z = #pixel
  
loop:
  y = zold
  zold = z
  z = z - (@coeffâ– â– z^@powerâ–  + @coeffâ– â– z^@powerâ–  - â– ) â–  @relax / \
          (@coeffâ– â– @powerâ– â– z^(@powerâ– -â– ) + @coeffâ– â– @powerâ– â– z^(@powerâ– -â– )) + @seed + @inductâ– y
  
bailout:
  |z - zold| > @bailout
  
default:
  title = "PhoenixDoubleNova (Julia)"
  helpfile = "dmj-pub\dmj-pub-uf-pdn.htm"
  maxiter = â– â– â– â– 
  periodicity = â– 
  center = (â– ,â– )
  magn = â– .â– 
  
  param seed
    caption = "Julia Seed"
    default = (â– ,â– )
    hint = "This is the Julia seed, a constant parameter which \
            defines the shape of the fractal."
  endparam
  param powerâ– 
    caption = "Primary Exponent"
    default = (â– ,â– )
    hint = "Defines the primary exponent for the equation."
  endparam
  param powerâ– 
    caption = "Secondary Exponent"
    default = (â– ,â– )
    hint = "Defines the secondary exponent for the equation."
  endparam
  param coeffâ– 
    caption = "Primary Scale"
    default = (â– ,â– )
    hint = "Defines the coefficient (multiplier) for the \
            primary exponent term."
  endparam
  param coeffâ– 
    caption = "Secondary Scale"
    default = (-â– ,â– )
    hint = "Defines the coefficient (multiplier) for the \
            secondary exponent term."
  endparam
  param induct
    caption = "Phoenix Distortion"
    default = (-â– .â– ,â– )
    hint = "Sets how 'strong' the previous iteration's effect should be \
            on the fractal."
  endparam
  param bailout
    caption = "Bailout"
    default = â– .â– â– â– â– â– 
    hint = "Bailout value; smaller values will cause more \
            iterations to be done for each point."
  endparam
  param relax
    caption = "Relaxation"
    default = (â– ,â– )
    hint = "This can be used to slow down the convergence of \
            the formula."
  endparam

switch:
  type = "dmj-PhoenixDNovaMandel"
  powerâ–  = @powerâ– 
  powerâ–  = @powerâ– 
  coeffâ–  = @coeffâ– 
  coeffâ–  = @coeffâ– 
  bailout = @bailout
  relax = @relax
}
not generated by AI logo

DIALOG series

OUTER SPACE series ELEMENTS series LIFE series DIALOG seriesBEYOND series

Fractal 153 B – AI Scanning Mankind
Fractal 153 B – AI Scanning Mankind
Fractal 152 A – Hello World !
Fractal 152 A – Hello World !
Fractal 146 C – Dance in the city
Fractal 146 C – Dance in the city
Fractal 144 A – Friend
Fractal 144 A – Friend
Fractal 143 B – Friend
Fractal 143 B – Friend
Fractal 123 F – Rembrandt’s Rich Woman Portrait
Fractal 123 F – Rembrandt’s Rich Woman Portrait
Fractal 121 B – Bonnard’s Light Trap
Fractal 121 B – Bonnard’s Light Trap
Fractal 119 A – Civilization
Fractal 119 A – Civilization
Fractal 098 E – Orgasm
Fractal 098 E – Orgasm
Fractal 113 B – Pixel Earth
Fractal 113 B – Pixel Earth
Fractal 112 B – Computer Emotion
Fractal 112 B – Computer Emotion
Fractal 105E – AI Scanning Mankind
Fractal 105E – AI Scanning Mankind
Fractal 100 E – Chip
Fractal 100 E – Chip
Fractal 099 A – Ego
Fractal 099 A – Ego
Fractal 095 B – Hopelessness
Fractal 095 B – Hopelessness
Fractal 092 C – Falling in Love
Fractal 092 C – Falling in Love
Fractal 087 D – Lust
Fractal 087 D – Lust
Fractal 056 C – Shyness
Fractal 056 C – Shyness
Fractal 014 C – Trip
Fractal 014 C – Trip