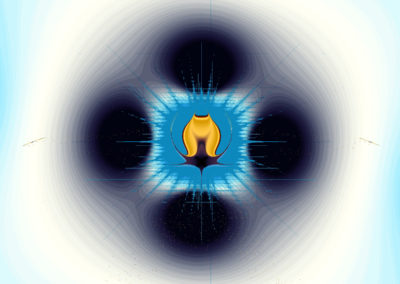
Fractal 143 B – Friend
Part of DIALOG series – 11 2023
dialogue . different . encounter . ET . other . stranger
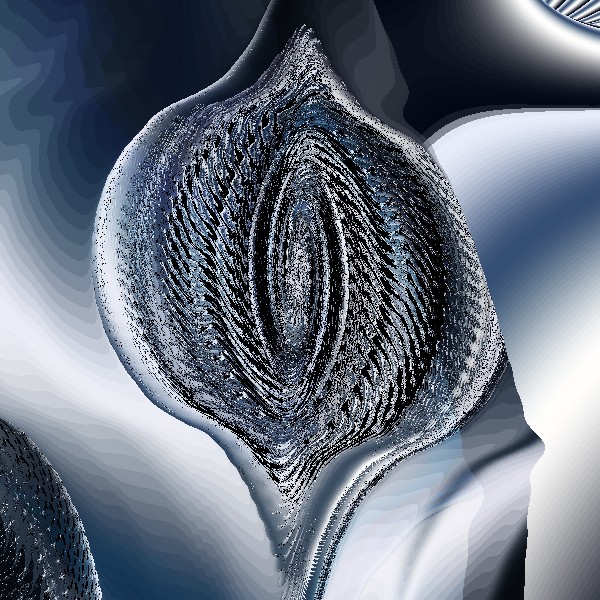
Fractal 143 B – zoom level 1
Fractal 143 B – zoom level 2
Data
This digital works is created exclusively from fractals, with 0% AI generated. It can therefore be converted into a set of functions and parameters that can be the basis for the training of an AI.
The mathematical formulas and parameter combinations corresponding to each fractal are presented below. In each case, the numerical characters have been replaced by â– to prevent unauthorized reproduction. If you are interested in using the full data set to train an AI, please contact Philippe.
Fractal 143 B - Friend - Part of DIALOG series - 11.2023
Fractal_â– â– â– _B {
fractal:
title="Fractal_â– â– â– _B" width=â– â– â– â– height=â– â– â– â– layers=â–
credits="Philoxerax;â– â– /â– /â– â– â– â– "
group:
caption="Head" opacity=â– â– â– mergemode=passthrough items=â–
layer:
caption="Layer â– " opacity=â– â– â– mergemode=multiply transparent=yes
mapping:
center=-â– .â– â– â– â– â– â– â– â– â– â– /â– .â– â– â– â– â– â– â– â– â– magn=â– .â– â– â– â– â– â– â– â– angle=â– â– â– .â– â– â– â–
stretch=â– .â– â– â– â– skew=-â– .â– â– â– â–
formula:
maxiter=â– â– â– filename="lkmâ– .ufm" entry="fourier-popcorn"
p_control_type=advanced p_bailout=â– â– p_hall=â– .â– /â–
p_functypeall="sin odd pos" p_powerall=â– /â– p_ntermall=â– â–
p_slopeall=â– /â– f_funcall=tan p_hx=â– .â– /â– p_vartypexin=y
p_functypexin="cos odd pos" p_powerxin=â– â– /â– p_ntermxin=â– â–
f_funcxin=cos p_vartypexout=y p_functypexout="cos odd pos"
p_powerxout=â– /â– p_ntermxout=â– â– p_slopexout=â– /â– f_funcxout=cos
p_hy=â– .â– /â– p_vartypeyin=x p_functypeyin="sin all pos"
p_poweryin=â– /â– .â– p_ntermyin=â– â– f_funcyin=sin p_vartypeyout=x
p_functypeyout="sin odd pos" p_poweryout=â– /â– p_ntermyout=â– â–
p_slopeyout=â– /â– f_funcyout=sin
inside:
transfer=none
outside:
transfer=linear
gradient:
smooth=yes rotation=-â– â– â– index=â– â– color=â– â– â– â– â– â– â– index=â– â– â–
color=â– â– â– â– â– â– â– â– index=â– â– â– color=â– â– â– â– â– â– â– index=â– â– â– color=â– â– â– â– â– â– â– â–
index=â– â– â– color=â– â– â– â– â– â– â– index=â– â– â– color=â– â– â– â– â– â– â– â–
opacity:
smooth=no index=â– opacity=â– index=â– â– opacity=â– â– â– index=â– â– â–
opacity=â– â– â–
layer:
caption="Layer â– " opacity=â– â– â– mergemode=screen transparent=yes
mapping:
center=-â– .â– â– â– â– â– â– â– â– â– â– /â– .â– â– â– â– â– â– â– â– â– magn=â– .â– â– â– â– â– â– â– â– angle=â– â– â– .â– â– â– â–
stretch=â– .â– â– â– â– skew=-â– .â– â– â– â–
formula:
maxiter=â– â– â– filename="lkmâ– .ufm" entry="fourier-popcorn"
p_control_type=advanced p_bailout=â– â– p_hall=â– .â– /â–
p_functypeall="sin odd pos" p_powerall=â– /â– p_ntermall=â– â–
p_slopeall=â– /â– f_funcall=tan p_hx=â– .â– /â– p_vartypexin=y
p_functypexin="cos general" p_powerxin=â– â– /â– p_ntermxin=â– â–
f_funcxin=cos p_vartypexout=y p_functypexout="cos odd pos"
p_powerxout=â– /â– p_ntermxout=â– â– p_slopexout=â– /â– f_funcxout=cos
p_hy=â– .â– /â– p_vartypeyin=x p_functypeyin="sin all pos" p_poweryin=â– /â–
p_ntermyin=â– â– f_funcyin=sin p_vartypeyout=x
p_functypeyout="sin odd pos" p_poweryout=â– /â– p_ntermyout=â– â–
p_slopeyout=â– /â– f_funcyout=sin
inside:
transfer=none
outside:
transfer=linear
gradient:
smooth=yes rotation=â– â– index=â– â– â– color=â– â– â– â– â– â– â– index=â– â– â–
color=â– â– â– â– â– â– â– index=â– â– â– color=â– â– â– â– â– â– â– index=â– â– â– color=â– â– â– â– â– â– â– â–
index=â– â– â– color=â– â– â– â– â– â– â– â– index=â– â– â– color=â– â– â– â– â– â– â– â– index=â– â– â–
color=â– â– â– â– â– â– â– â– index=â– â– â– color=â– â– â– â– â– â– â– â– index=â– â– â– color=â– â– â– â– â– â– â– â–
index=â– â– â– color=â– â– â– â– â– â– â– â– index=â– â– â– color=â– â– â– â– â– â– â– â– index=â– â– â–
color=â– â– â– â– â– â– â– index=â– â– â– color=â– â– â– â– â– â– â– index=â– â– â– color=â– â– â– â– â– â– â– â–
index=â– â– â– color=â– â– â– â– â– â– â– index=â– â– â– color=â– â– â– â– â– â– â– â– index=â– â– â–
color=â– â– â– â– â– â– â– â– index=â– â– â– color=â– â– â– â– â– â– â– â– index=â– â– â– color=â– â– â– â– â– â– â– â–
index=â– â– â– color=â– â– â– â– â– â– â–
opacity:
smooth=no index=â– opacity=â– index=â– â– opacity=â– â– â– index=â– â– â–
opacity=â– â– â–
layer:
caption="Layer â– " opacity=â– â– â– transparent=yes
mapping:
center=-â– .â– â– â– â– â– â– â– â– â– â– /â– .â– â– â– â– â– â– â– â– â– magn=â– .â– â– â– â– â– â– â– â– angle=â– â– â– .â– â– â– â–
stretch=â– .â– â– â– â– skew=-â– .â– â– â– â–
formula:
maxiter=â– â– â– filename="lkmâ– .ufm" entry="fourier-popcorn"
p_control_type=advanced p_bailout=â– â– p_hall=â– .â– /â–
p_functypeall="sin odd pos" p_powerall=â– /â– p_ntermall=â– â–
p_slopeall=â– /â– f_funcall=tan p_hx=â– .â– /â– p_vartypexin=y
p_functypexin="cos odd pos" p_powerxin=â– â– /â– p_ntermxin=â– â–
f_funcxin=cos p_vartypexout=y p_functypexout="cos odd pos"
p_powerxout=â– /â– p_ntermxout=â– â– p_slopexout=â– /â– f_funcxout=cos
p_hy=â– .â– /â– p_vartypeyin=x p_functypeyin="sin all pos"
p_poweryin=â– /â– .â– p_ntermyin=â– â– f_funcyin=sin p_vartypeyout=x
p_functypeyout="sin odd pos" p_poweryout=â– /â– p_ntermyout=â– â–
p_slopeyout=â– /â– f_funcyout=sin
inside:
transfer=none
outside:
transfer=linear
gradient:
smooth=yes rotation=-â– â– â– index=â– â– color=â– â– â– â– â– â– â– index=â– â– â–
color=â– â– â– â– â– â– â– â– index=â– â– â– color=â– â– â– â– â– â– â– index=â– â– â– color=â– â– â– â– â– â– â– â–
index=â– â– â– color=â– â– â– â– â– â– â– index=â– â– â– color=â– â– â– â– â– â– â– â–
opacity:
smooth=no index=â– opacity=â– index=â– â– opacity=â– â– â– index=â– â– â–
opacity=â– â– â–
group:
caption="Background" opacity=â– â– â– mergemode=passthrough items=â–
layer:
caption="Layer â– " opacity=â– â– visible=no mergemode=multiply
mapping:
center=-â– .â– â– â– â– â– â– â– â– â– â– â– /-â– .â– â– â– â– â– â– â– â– â– magn=â– .â– â– â– â– â– â– â– angle=â– â– â– .â– â– â–
formula:
maxiter=â– â– â– â– percheck=off filename="mt.ufm"
entry="mt-bof-newton-I-m" p_s=â– â– â– .â– p_epsilon=â– E-â–
inside:
transfer=none
outside:
transfer=linear
gradient:
smooth=yes index=â– color=â– â– â– â– â– â– â– â– index=â– color=â– â– â– â– â– â– â– index=â–
color=â– â– â– â– â– â– index=â– color=â– â– â– â– â– â– â– index=â– color=â– â– â– â– â– â– â– index=â–
color=â– â– â– â– â– â– â– index=â– color=â– â– â– â– â– â– â– index=â– color=â– â– â– â– â– â– â– index=â–
color=â– â– â– â– â– â– â– index=â– color=â– â– â– â– â– â– â– â– index=â– â– color=â– â– â– â– â– â– â– â–
index=â– â– color=â– â– â– â– â– â– â– â– index=â– â– color=â– â– â– â– â– â– â– index=â– â– color=â– â– â– â– â– â–
index=â– â– color=â– â– â– â– â– â– â– index=â– â– color=â– â– â– â– â– â– â– â– index=â– â–
color=â– â– â– â– â– â– â– â– index=â– â– color=â– â– â– â– â– â– index=â– â– color=â– â– â– â– â– â– â– index=â– â–
color=â– â– â– â– â– â– â– index=â– â– color=â– â– â– â– â– â– â– index=â– â– color=â– â– â– â– â– â– â– â–
index=â– â– color=â– â– â– â– â– â– â– index=â– â– color=â– â– â– â– â– â– â– index=â– â–
color=â– â– â– â– â– â– â– â– index=â– â– color=â– â– â– â– â– â– â– index=â– â– color=â– â– â– â– â– â– â–
index=â– â– color=â– â– â– â– â– â– â– index=â– â– color=â– â– â– â– â– â– index=â– â– color=â– â– â– â– â– â– â–
index=â– â– color=â– â– â– â– â– â– â– â– index=â– â– color=â– â– â– â– â– â– index=â– â–
color=â– â– â– â– â– â– â– â– index=â– â– color=â– â– â– â– â– â– â– â– index=â– â– color=â– â– â– â– â– â– â–
index=â– â– color=â– â– â– â– â– â– â– index=â– â– color=â– â– â– â– â– â– â– index=â– â–
color=â– â– â– â– â– â– â– â– index=â– â– color=â– â– â– â– â– â– â– index=â– â– color=â– â– â– â– â– â– index=â– â–
color=â– â– â– â– â– â– â– index=â– â– color=â– â– â– â– â– â– â– index=â– â– color=â– â– â– â– â– â– â– â–
index=â– â– color=â– â– â– â– â– â– â– index=â– â– color=â– â– â– â– â– â– â– index=â– â–
color=â– â– â– â– â– â– â– â– index=â– â– color=â– â– â– â– â– â– â– â– index=â– â– color=â– â– â– â– â– â– â– â–
index=â– â– color=â– â– â– â– â– â– â– index=â– â– color=â– â– â– â– â– â– â– â– index=â– â–
color=â– â– â– â– â– â– â– â– index=â– â– color=â– â– â– â– â– â– â– â– index=â– â– color=â– â– â– â– â– â– â–
index=â– â– color=â– â– â– â– â– â– â– â– index=â– â– color=â– â– â– â– â– â– â– â– index=â– â–
color=â– â– â– â– â– â– â– index=â– â– color=â– â– â– â– â– â– â– â– index=â– â– color=â– â– â– â– â– â– â– â–
index=â– â– color=â– â– â– â– â– â– â– â– index=â– â– color=â– â– â– â– â– â– â– â– index=â– â–
color=â– â– â– â– â– â– â– index=â– â– color=â– â– â– â– â– â– â– index=â– â– color=â– â– â– â– â– â– â– â–
index=â– â– color=â– â– â– â– â– â– â– index=â– â– color=â– â– â– â– â– â– â– index=â– â– color=â– â– â– â– â– â– â–
index=â– â– color=â– â– â– â– â– â– â– â– index=â– â– color=â– â– â– â– â– â– â– â– index=â– â–
color=â– â– â– â– â– â– â– index=â– â– color=â– â– â– â– â– â– index=â– â– â– color=â– â– â– â– â– â– â– â–
index=â– â– â– color=â– â– â– â– â– â– â– index=â– â– â– color=â– â– â– â– â– â– â– â– index=â– â– â–
color=â– â– â– â– â– â– â– â– index=â– â– â– color=â– â– â– â– â– â– â– â– index=â– â– â– color=â– â– â– â– â– â– â–
index=â– â– â– color=â– â– â– â– â– â– â– â– index=â– â– â– color=â– â– â– â– â– â– â– index=â– â– â–
color=â– â– â– â– â– â– â– index=â– â– â– color=â– â– â– â– â– â– â– â– index=â– â– â– color=â– â– â– â– â– â– â–
index=â– â– â– color=â– â– â– â– â– â– â– â– index=â– â– â– color=â– â– â– â– â– â– â– â– index=â– â– â–
color=â– â– â– â– â– â– â– index=â– â– â– color=â– â– â– â– â– â– â– index=â– â– â– color=â– â– â– â– â– â– â–
index=â– â– â– color=â– â– â– â– â– â– â– index=â– â– â– color=â– â– â– â– â– â– â– index=â– â– â–
color=â– â– â– â– â– â– â– index=â– â– â– color=â– â– â– â– â– â– â– â– index=â– â– â– color=â– â– â– â– â– â– â– â–
index=â– â– â– color=â– â– â– â– â– â– â– â– index=â– â– â– color=â– â– â– â– â– â– â– â– index=â– â– â–
color=â– â– â– â– â– â– â– index=â– â– â– color=â– â– â– â– â– â– â– index=â– â– â– color=â– â– â– â– â– â– â– â–
index=â– â– â– color=â– â– â– â– â– â– â– â– index=â– â– â– color=â– â– â– â– â– â– â– index=â– â– â–
color=â– â– â– â– â– â– â– index=â– â– â– color=â– â– â– â– â– â– â– index=â– â– â– color=â– â– â– â– â– â– â– â–
index=â– â– â– color=â– â– â– â– â– â– â– index=â– â– â– color=â– â– â– â– â– â– â– â– index=â– â– â–
color=â– â– â– â– â– â– â– index=â– â– â– color=â– â– â– â– â– â– â– index=â– â– â– color=â– â– â– â– â– â– â– â–
index=â– â– â– color=â– â– â– â– â– â– â– â– index=â– â– â– color=â– â– â– â– â– â– â– index=â– â– â–
color=â– â– â– â– â– â– â– â– index=â– â– â– color=â– â– â– â– â– â– â– â– index=â– â– â– color=â– â– â– â– â– â– â–
index=â– â– â– color=â– â– â– â– â– â– â– â– index=â– â– â– color=â– â– â– â– â– â– â– â– index=â– â– â–
color=â– â– â– â– â– â– â– index=â– â– â– color=â– â– â– â– â– â– index=â– â– â– color=â– â– â– â– â– â– â– â–
index=â– â– â– color=â– â– â– â– â– â– â– â– index=â– â– â– color=â– â– â– â– â– â– â– â– index=â– â– â–
color=â– â– â– â– â– â– â– â– index=â– â– â– color=â– â– â– â– â– â– â– index=â– â– â– color=â– â– â– â– â– â– â–
index=â– â– â– color=â– â– â– â– â– â– index=â– â– â– color=â– â– â– â– â– â– â– â– index=â– â– â–
color=â– â– â– â– â– â– â– â– index=â– â– â– color=â– â– â– â– â– â– â– â– index=â– â– â– color=â– â– â– â– â– â– â– â–
index=â– â– â– color=â– â– â– â– â– â– â– â– index=â– â– â– color=â– â– â– â– â– â– â– â– index=â– â– â–
color=â– â– â– â– â– â– â– index=â– â– â– color=â– â– â– â– â– â– index=â– â– â– color=â– â– â– â– â– â– â– â–
index=â– â– â– color=â– â– â– â– â– â– â– index=â– â– â– color=â– â– â– â– â– â– â– index=â– â– â–
color=â– â– â– â– â– â– â– index=â– â– â– color=â– â– â– â– â– â– â– â– index=â– â– â– color=â– â– â– â– â– â– â–
index=â– â– â– color=â– â– â– â– â– â– â– index=â– â– â– color=â– â– â– â– â– â– â– index=â– â– â–
color=â– â– â– â– â– â– â– â– index=â– â– â– color=â– â– â– â– â– â– â– â– index=â– â– â– color=â– â– â– â– â– â– â–
index=â– â– â– color=â– â– â– â– â– â– â– â– index=â– â– â– color=â– â– â– â– â– â– â– index=â– â– â–
color=â– â– â– â– â– â– â– â– index=â– â– â– color=â– â– â– â– â– â– â– index=â– â– â– color=â– â– â– â– â– â– â–
index=â– â– â– color=â– â– â– â– â– â– â– index=â– â– â– color=â– â– â– â– â– â– â– index=â– â– â–
color=â– â– â– â– â– â– index=â– â– â– color=â– â– â– â– â– â– â– index=â– â– â– color=â– â– â– â– â– â–
index=â– â– â– color=â– â– â– â– â– â– â– index=â– â– â– color=â– â– â– â– â– â– â– â– index=â– â– â–
color=â– â– â– â– â– â– â– â– index=â– â– â– color=â– â– â– â– â– â– â– index=â– â– â– color=â– â– â– â– â– â– â–
index=â– â– â– color=â– â– â– â– â– â– â– â– index=â– â– â– color=â– â– â– â– â– â– â– index=â– â– â–
color=â– â– â– â– â– â– â– index=â– â– â– color=â– â– â– â– â– â– â– â– index=â– â– â– color=â– â– â– â– â– â– â– â–
index=â– â– â– color=â– â– â– â– â– â– â– â– index=â– â– â– color=â– â– â– â– â– â– â– index=â– â– â–
color=â– â– â– â– â– â– â– â– index=â– â– â– color=â– â– â– â– â– â– â– â– index=â– â– â– color=â– â– â– â– â– â– â– â–
index=â– â– â– color=â– â– â– â– â– â– â– index=â– â– â– color=â– â– â– â– â– â– â– index=â– â– â–
color=â– â– â– â– â– â– index=â– â– â– color=â– â– â– â– â– â– â– index=â– â– â– color=â– â– â– â– â– â– â–
index=â– â– â– color=â– â– â– â– â– â– â– â– index=â– â– â– color=â– â– â– â– â– â– â– index=â– â– â–
color=â– â– â– â– â– â– â– index=â– â– â– color=â– â– â– â– â– â– â– index=â– â– â– color=â– â– â– â– â– â– â– â–
index=â– â– â– color=â– â– â– â– â– â– â– index=â– â– â– color=â– â– â– â– â– â– â– index=â– â– â–
color=â– â– â– â– â– â– index=â– â– â– color=â– â– â– â– â– â– â– index=â– â– â– color=â– â– â– â– â– â– â–
index=â– â– â– color=â– â– â– â– â– â– â– â– index=â– â– â– color=â– â– â– â– â– â– â– â– index=â– â– â–
color=â– â– â– â– â– â– â– â– index=â– â– â– color=â– â– â– â– â– â– â– â– index=â– â– â– color=â– â– â– â– â– â– â– â–
index=â– â– â– color=â– â– â– â– â– â– â– index=â– â– â– color=â– â– â– â– â– â– â– â– index=â– â– â–
color=â– â– â– â– â– â– â– index=â– â– â– color=â– â– â– â– â– â– â– â– index=â– â– â– color=â– â– â– â– â– â–
index=â– â– â– color=â– â– â– â– â– â– â– index=â– â– â– color=â– â– â– â– â– â– â– â– index=â– â– â–
color=â– â– â– â– â– â– â– â– index=â– â– â– color=â– â– â– â– â– â– â– index=â– â– â– color=â– â– â– â– â– â– â–
index=â– â– â– color=â– â– â– â– â– â– â– index=â– â– â– color=â– â– â– â– â– â– â– index=â– â– â–
color=â– â– â– â– â– â– â– â– index=â– â– â– color=â– â– â– â– â– â– â– index=â– â– â– color=â– â– â– â– â– â– â– â–
index=â– â– â– color=â– â– â– â– â– â– â– â– index=â– â– â– color=â– â– â– â– â– â– â– index=â– â– â–
color=â– â– â– â– â– â– â– index=â– â– â– color=â– â– â– â– â– â– â– â– index=â– â– â– color=â– â– â– â– â– â– â–
index=â– â– â– color=â– â– â– â– â– â– â– index=â– â– â– color=â– â– â– â– â– â– â– â– index=â– â– â–
color=â– â– â– â– â– â– â– index=â– â– â– color=â– â– â– â– â– â– â– index=â– â– â– color=â– â– â– â– â– â– â–
index=â– â– â– color=â– â– â– â– â– â– â– â– index=â– â– â– color=â– â– â– â– â– â– â– index=â– â– â–
color=â– â– â– â– â– â– â– index=â– â– â– color=â– â– â– â– â– â– â– â– index=â– â– â– color=â– â– â– â– â– â– â– â–
index=â– â– â– color=â– â– â– â– â– â– â– index=â– â– â– color=â– â– â– â– â– â– index=â– â– â–
color=â– â– â– â– â– â– â– index=â– â– â– color=â– â– â– â– â– â– â–
opacity:
smooth=no index=â– opacity=â– â– â–
layer:
caption="Background" opacity=â– â– â–
mapping:
center=-â– .â– â– â– â– â– â– â– â– â– â– â– /-â– .â– â– â– â– â– â– â– â– â– magn=â– .â– â– â– â– â– â– â– angle=â– â– â– .â– â– â– â–
formula:
maxiter=â– â– â– â– percheck=off filename="mt.ufm"
entry="mt-bof-newton-I-m" p_s=â– â– â– .â– p_epsilon=â– E-â–
inside:
transfer=none solid=â– â– â– â– â– â– â– â– â– â–
outside:
transfer=linear
gradient:
smooth=yes index=â– â– color=â– â– â– â– â– â– â– index=â– â– â– color=â– â– â– â– â– â– â– â– index=â– â– â–
color=â– â– â– â– â– â– â– index=â– â– â– color=â– â– â– â– â– â– â– index=â– â– â– color=â– â– â– â– â– â– â– â–
opacity:
smooth=no index=â– opacity=â– â– â–
layer:
caption="Layer â– " opacity=â– â– â– transparent=yes mask=yes
mapping:
center=-â– .â– â– â– â– â– â– â– â– â– â– â– /-â– .â– â– â– â– â– â– â– â– â– magn=â– .â– â– â– â– â– â– â– angle=â– â– â– .â– â– â– â–
formula:
maxiter=â– â– â– â– percheck=off filename="mt.ufm"
entry="mt-bof-newton-I-m" p_s=â– â– â– .â– p_epsilon=â– E-â–
inside:
transfer=none solid=â–
outside:
transfer=linear
gradient:
smooth=yes index=â– â– color=â– â– â– â– â– â– â– index=â– â– â– color=â– â– â– â– â– â– â– â– index=â– â– â–
color=â– â– â– â– â– â– â– index=â– â– â– color=â– â– â– â– â– â– â– â–
opacity:
smooth=no index=â– opacity=â– â– â–
layer:
caption="Layer â– " opacity=â– â– â–
mapping:
center=-â– .â– â– â– â– â– â– â– â– â– â– /â– .â– â– â– â– â– â– â– â– â– â– magn=â– .â– â– â– â– â– â– â– angle=â– â– â– .â– â– â– â–
formula:
maxiter=â– â– â– â– percheck=off filename="mt.ufm"
entry="mt-bof-newton-I-m" p_s=â– â– â– .â– p_epsilon=â– E-â–
inside:
transfer=none solid=â– â– â– â– â– â– â– â– â– â–
outside:
transfer=linear
gradient:
smooth=yes index=â– â– color=â– â– â– â– â– â– â– index=â– â– â– color=â– â– â– â– â– â– â– â– index=â– â– â–
color=â– â– â– â– â– â– â– index=â– â– â– color=â– â– â– â– â– â– â– â–
opacity:
smooth=no index=â– opacity=â– â– â–
}
fourier-popcorn { ; Kerry Mitchell â– â– Mayâ– â– â– â–
;
; This is a complex-valued implementation and generalization
; of Cliff Pickover's "popcorn" algorithm. His method iterates
; â– real variables like this:
;
; xnew = xold - h â– sin(aâ– y + tan(bâ– y))
; ynew = yold - h â– sin(aâ– x + tan(bâ– x))
;
; where a = â– . The current method generalizes the above by:
; using truncated Fourier series instead of just single sin or tan function
; allowing for complex values of a (slope)
; having the same Fourier series parameters (basic controls) or
; having different parameters for the â– series (x inner, x outer,
; y inner, and y outer)
;
init:
complex temp=(â– ,â– )
complex tempx=(â– ,â– )
complex tempy=(â– ,â– )
complex tempâ– =(â– ,â– )
complex tempâ– x=(â– ,â– )
complex tempâ– y=(â– ,â– )
complex tempin=(â– ,â– )
complex tempinx=(â– ,â– )
complex tempiny=(â– ,â– )
complex tempout=(â– ,â– )
complex tempoutx=(â– ,â– )
complex tempouty=(â– ,â– )
complex x=real(#pixel)
complex y=imag(#pixel)
complex xnew=(â– ,â– )
complex ynew=(â– ,â– )
complex z=#pixel
int iterm=â–
int jterm=â–
int parity=â–
loop:
;
; basic controls
;
if(@control_type=="basic")
;
; choose what inner variable to use
;
tempinx=y
tempiny=x
;
; compute the inner Fourier series
;
tempx=â–
tempy=â–
iterm=â–
while(iterm<@ntermall)
iterm=iterm+â–
if(@functypeall=="sin odd pos")
jterm=â– â– iterm-â–
tempx=tempx+sin(tempinxâ– jterm)/(jterm^@powerall)
tempy=tempy+sin(tempinyâ– jterm)/(jterm^@powerall)
elseif(@functypeall=="cos odd pos")
jterm=â– â– iterm-â–
tempx=tempx+cos(tempinxâ– jterm)/(jterm^@powerall)
tempy=tempy+cos(tempinyâ– jterm)/(jterm^@powerall)
elseif(@functypeall=="sin all pos")
jterm=iterm
tempx=tempx+sin(tempinxâ– jterm)/(jterm^@powerall)
tempy=tempy+sin(tempinyâ– jterm)/(jterm^@powerall)
elseif(@functypeall=="cos all pos")
jterm=iterm
tempx=tempx+cos(tempinxâ– jterm)/(jterm^@powerall)
tempy=tempy+cos(tempinyâ– jterm)/(jterm^@powerall)
elseif(@functypeall=="sin odd alt")
jterm=â– â– iterm-â–
parity=(iterm%â– )â– â– -â–
tempx=tempx+parityâ– sin(tempinxâ– jterm)/(jterm^@powerall)
tempy=tempy+parityâ– sin(tempinyâ– jterm)/(jterm^@powerall)
elseif(@functypeall=="cos odd alt")
jterm=â– â– iterm-â–
parity=(iterm%â– )â– â– -â–
tempx=tempx+parityâ– cos(tempinxâ– jterm)/(jterm^@powerall)
tempy=tempy+parityâ– cos(tempinyâ– jterm)/(jterm^@powerall)
elseif(@functypeall=="sin all alt")
jterm=iterm
parity=(iterm%â– )â– â– -â–
tempx=tempx+parityâ– sin(tempinxâ– jterm)/(jterm^@powerall)
tempy=tempy+parityâ– sin(tempinyâ– jterm)/(jterm^@powerall)
elseif(@functypeall=="cos all alt")
jterm=iterm
parity=(iterm%â– )â– â– -â–
tempx=tempx+parityâ– cos(tempinxâ– jterm)/(jterm^@powerall)
tempy=tempy+parityâ– cos(tempinyâ– jterm)/(jterm^@powerall)
elseif(@functypeall=="sin general")
jterm=iterm
tempx=tempx+@funcall(jterm)â– sin(tempinxâ– jterm)/(jterm^@powerall)
tempy=tempy+@funcall(jterm)â– sin(tempinyâ– jterm)/(jterm^@powerall)
elseif(@functypeall=="cos general")
jterm=iterm
tempx=tempx+@funcall(jterm)â– cos(tempinxâ– jterm)/(jterm^@powerall)
tempy=tempy+@funcall(jterm)â– cos(tempinyâ– jterm)/(jterm^@powerall)
endif
endwhile
;
; choose what outer variable to use
;
tempoutx=y
tempouty=x
;
; compute the outer Fourier series
;
tempâ– x=â–
tempâ– y=â–
iterm=â–
while(iterm<@ntermall)
iterm=iterm+â–
if(@functypeall=="sin odd pos")
jterm=â– â– iterm-â–
tempâ– x=tempâ– x+sin((@slopeallâ– tempoutx+tempx)â– jterm)/(jterm^@powerall)
tempâ– y=tempâ– y+sin((@slopeallâ– tempouty+tempy)â– jterm)/(jterm^@powerall)
elseif(@functypeall=="cos odd pos")
jterm=â– â– iterm-â–
tempâ– x=tempâ– x+cos((@slopeallâ– tempoutx+tempx)â– jterm)/(jterm^@powerall)
tempâ– y=tempâ– y+cos((@slopeallâ– tempouty+tempy)â– jterm)/(jterm^@powerall)
elseif(@functypeall=="sin all pos")
jterm=iterm
tempâ– x=tempâ– x+sin((@slopeallâ– tempoutx+tempx)â– jterm)/(jterm^@powerall)
tempâ– y=tempâ– y+sin((@slopeallâ– tempouty+tempy)â– jterm)/(jterm^@powerall)
elseif(@functypeall=="cos all pos")
jterm=iterm
tempâ– x=tempâ– x+cos((@slopeallâ– tempoutx+tempx)â– jterm)/(jterm^@powerall)
tempâ– y=tempâ– y+cos((@slopeallâ– tempouty+tempy)â– jterm)/(jterm^@powerall)
elseif(@functypeall=="sin odd alt")
jterm=â– â– iterm-â–
parity=(iterm%â– )â– â– -â–
tempâ– x=tempâ– x+parityâ– sin((@slopeallâ– tempoutx+tempx)â– jterm)/(jterm^@powerall)
tempâ– y=tempâ– y+parityâ– sin((@slopeallâ– tempouty+tempy)â– jterm)/(jterm^@powerall)
elseif(@functypeall=="cos odd alt")
jterm=â– â– iterm-â–
parity=(iterm%â– )â– â– -â–
tempâ– x=tempâ– x+parityâ– cos((@slopeallâ– tempoutx+tempx)â– jterm)/(jterm^@powerall)
tempâ– y=tempâ– y+parityâ– cos((@slopeallâ– tempouty+tempy)â– jterm)/(jterm^@powerall)
elseif(@functypeall=="sin all alt")
jterm=iterm
parity=(iterm%â– )â– â– -â–
tempâ– x=tempâ– x+parityâ– sin((@slopeallâ– tempoutx+tempx)â– jterm)/(jterm^@powerall)
tempâ– y=tempâ– y+parityâ– sin((@slopeallâ– tempouty+tempy)â– jterm)/(jterm^@powerall)
elseif(@functypeall=="cos all alt")
jterm=iterm
parity=(iterm%â– )â– â– -â–
tempâ– x=tempâ– x+parityâ– cos((@slopeallâ– tempoutx+tempx)â– jterm)/(jterm^@powerall)
tempâ– y=tempâ– y+parityâ– cos((@slopeallâ– tempouty+tempy)â– jterm)/(jterm^@powerall)
elseif(@functypeall=="sin general")
jterm=iterm
tempâ– x=tempâ– x+@funcall(jterm)â– sin((@slopeallâ– tempoutx+tempx)â– jterm)/(jterm^@powerall)
tempâ– y=tempâ– y+@funcall(jterm)â– sin((@slopeallâ– tempouty+tempy)â– jterm)/(jterm^@powerall)
elseif(@functypeall=="cos general")
jterm=iterm
tempâ– x=tempâ– x+@funcall(jterm)â– cos((@slopeallâ– tempoutx+tempx)â– jterm)/(jterm^@powerall)
tempâ– y=tempâ– y+@funcall(jterm)â– cos((@slopeallâ– tempouty+tempy)â– jterm)/(jterm^@powerall)
endif
endwhile
xnew=x+@hallâ– tempâ– x
ynew=y+@hallâ– tempâ– y
x=xnew
y=ynew
z=x+flip(y)
;
; advanced controls
;
else
;
; x equation
;
; choose what inner variable to use
;
if(@vartypexin=="x")
tempin=x
elseif(@vartypexin=="y")
tempin=y
elseif(@vartypexin=="x+y")
tempin=x+y
elseif(@vartypexin=="y-x")
tempin=y-x
elseif(@vartypexin=="xâ– y")
tempin=xâ– y
elseif(@vartypexin=="y/x")
tempin=y/x
endif
;
; compute the inner Fourier series
;
temp=â–
iterm=â–
while(iterm<@ntermxin)
iterm=iterm+â–
if(@functypexin=="sin odd pos")
jterm=â– â– iterm-â–
temp=temp+sin(tempinâ– jterm)/(jterm^@powerxin)
elseif(@functypexin=="cos odd pos")
jterm=â– â– iterm-â–
temp=temp+cos(tempinâ– jterm)/(jterm^@powerxin)
elseif(@functypexin=="sin all pos")
jterm=iterm
temp=temp+sin(tempinâ– jterm)/(jterm^@powerxin)
elseif(@functypexin=="cos all pos")
jterm=iterm
temp=temp+cos(tempinâ– jterm)/(jterm^@powerxin)
elseif(@functypexin=="sin odd alt")
jterm=â– â– iterm-â–
parity=(iterm%â– )â– â– -â–
temp=temp+parityâ– sin(tempinâ– jterm)/(jterm^@powerxin)
elseif(@functypexin=="cos odd alt")
jterm=â– â– iterm-â–
parity=(iterm%â– )â– â– -â–
temp=temp+parityâ– cos(tempinâ– jterm)/(jterm^@powerxin)
elseif(@functypexin=="sin all alt")
jterm=iterm
parity=(iterm%â– )â– â– -â–
temp=temp+parityâ– sin(tempinâ– jterm)/(jterm^@powerxin)
elseif(@functypexin=="cos all alt")
jterm=iterm
parity=(iterm%â– )â– â– -â–
temp=temp+parityâ– cos(tempinâ– jterm)/(jterm^@powerxin)
elseif(@functypexin=="sin general")
jterm=iterm
temp=temp+@funcxin(jterm)â– sin(tempinâ– jterm)/(jterm^@powerxin)
elseif(@functypexin=="cos general")
jterm=iterm
temp=temp+@funcxin(jterm)â– cos(tempinâ– jterm)/(jterm^@powerxin)
endif
endwhile
;
; choose what outer variable to use
;
if(@vartypexout=="x")
tempout=x
elseif(@vartypexout=="y")
tempout=y
elseif(@vartypexout=="x+y")
tempout=x+y
elseif(@vartypexout=="y-x")
tempout=y-x
elseif(@vartypexout=="xâ– y")
tempout=xâ– y
elseif(@vartypexout=="y/x")
tempout=y/x
endif
;
; compute the outer Fourier series
;
tempâ– =â–
iterm=â–
while(iterm<@ntermxout)
iterm=iterm+â–
if(@functypexout=="sin odd pos")
jterm=â– â– iterm-â–
tempâ– =tempâ– +sin((@slopexoutâ– tempout+temp)â– jterm)/(jterm^@powerxout)
elseif(@functypexout=="cos odd pos")
jterm=â– â– iterm-â–
tempâ– =tempâ– +cos((@slopexoutâ– tempout+temp)â– jterm)/(jterm^@powerxout)
elseif(@functypexout=="sin all pos")
jterm=iterm
tempâ– =tempâ– +sin((@slopexoutâ– tempout+temp)â– jterm)/(jterm^@powerxout)
elseif(@functypexout=="cos all pos")
jterm=iterm
tempâ– =tempâ– +cos((@slopexoutâ– tempout+temp)â– jterm)/(jterm^@powerxout)
elseif(@functypexout=="sin odd alt")
jterm=â– â– iterm-â–
parity=(iterm%â– )â– â– -â–
tempâ– =tempâ– +parityâ– sin((@slopexoutâ– tempout+temp)â– jterm)/(jterm^@powerxout)
elseif(@functypexout=="cos odd alt")
jterm=â– â– iterm-â–
parity=(iterm%â– )â– â– -â–
tempâ– =tempâ– +parityâ– cos((@slopexoutâ– tempout+temp)â– jterm)/(jterm^@powerxout)
elseif(@functypexout=="sin all alt")
jterm=iterm
parity=(iterm%â– )â– â– -â–
tempâ– =tempâ– +parityâ– sin((@slopexoutâ– tempout+temp)â– jterm)/(jterm^@powerxout)
elseif(@functypexout=="cos all alt")
jterm=iterm
parity=(iterm%â– )â– â– -â–
tempâ– =tempâ– +parityâ– cos((@slopexoutâ– tempout+temp)â– jterm)/(jterm^@powerxout)
elseif(@functypexout=="sin general")
jterm=iterm
tempâ– =tempâ– +@funcxout(jterm)â– sin((@slopexoutâ– tempout+temp)â– jterm)/(jterm^@powerxout)
elseif(@functypexout=="cos general")
jterm=iterm
tempâ– =tempâ– +@funcxout(jterm)â– cos((@slopexoutâ– tempout+temp)â– jterm)/(jterm^@powerxout)
endif
endwhile
xnew=x+@hxâ– tempâ–
;
; y equation
;
; choose what inner variable to use
;
if(@vartypeyin=="x")
tempin=x
elseif(@vartypeyin=="y")
tempin=y
elseif(@vartypeyin=="x+y")
tempin=x+y
elseif(@vartypeyin=="y-x")
tempin=y-x
elseif(@vartypeyin=="xâ– y")
tempin=xâ– y
elseif(@vartypeyin=="y/x")
tempin=y/x
endif
;
; compute the inner Fourier series
;
temp=â–
iterm=â–
while(iterm<@ntermyin)
iterm=iterm+â–
if(@functypeyin=="sin odd pos")
jterm=â– â– iterm-â–
temp=temp+sin(tempinâ– jterm)/(jterm^@poweryin)
elseif(@functypeyin=="cos odd pos")
jterm=â– â– iterm-â–
temp=temp+cos(tempinâ– jterm)/(jterm^@poweryin)
elseif(@functypeyin=="sin all pos")
jterm=iterm
temp=temp+sin(tempinâ– jterm)/(jterm^@poweryin)
elseif(@functypeyin=="cos all pos")
jterm=iterm
temp=temp+cos(tempinâ– jterm)/(jterm^@poweryin)
elseif(@functypeyin=="sin odd alt")
jterm=â– â– iterm-â–
parity=(iterm%â– )â– â– -â–
temp=temp+parityâ– sin(tempinâ– jterm)/(jterm^@poweryin)
elseif(@functypeyin=="cos odd alt")
jterm=â– â– iterm-â–
parity=(iterm%â– )â– â– -â–
temp=temp+parityâ– cos(tempinâ– jterm)/(jterm^@poweryin)
elseif(@functypeyin=="sin all alt")
jterm=iterm
parity=(iterm%â– )â– â– -â–
temp=temp+parityâ– sin(tempinâ– jterm)/(jterm^@poweryin)
elseif(@functypeyin=="cos all alt")
jterm=iterm
parity=(iterm%â– )â– â– -â–
temp=temp+parityâ– cos(tempinâ– jterm)/(jterm^@poweryin)
elseif(@functypeyin=="sin general")
jterm=iterm
temp=temp+@funcyin(jterm)â– sin(tempinâ– jterm)/(jterm^@poweryin)
elseif(@functypeyin=="cos general")
jterm=iterm
temp=temp+@funcyin(jterm)â– cos(tempinâ– jterm)/(jterm^@poweryin)
endif
endwhile
;
; choose what outer variable to use
;
if(@vartypeyout=="x")
tempout=x
elseif(@vartypeyout=="y")
tempout=y
elseif(@vartypeyout=="x+y")
tempout=x+y
elseif(@vartypeyout=="y-x")
tempout=y-x
elseif(@vartypeyout=="xâ– y")
tempout=xâ– y
elseif(@vartypeyout=="y/x")
tempout=y/x
endif
;
; compute the outer Fourier series
;
tempâ– =â–
iterm=â–
while(iterm<@ntermyout)
iterm=iterm+â–
if(@functypeyout=="sin odd pos")
jterm=â– â– iterm-â–
tempâ– =tempâ– +sin((@slopeyoutâ– tempout+temp)â– jterm)/(jterm^@poweryout)
elseif(@functypeyout=="cos odd pos")
jterm=â– â– iterm-â–
tempâ– =tempâ– +cos((@slopeyoutâ– tempout+temp)â– jterm)/(jterm^@poweryout)
elseif(@functypeyout=="sin all pos")
jterm=iterm
tempâ– =tempâ– +sin((@slopeyoutâ– tempout+temp)â– jterm)/(jterm^@poweryout)
elseif(@functypeyout=="cos all pos")
jterm=iterm
tempâ– =tempâ– +cos((@slopeyoutâ– tempout+temp)â– jterm)/(jterm^@poweryout)
elseif(@functypeyout=="sin odd alt")
jterm=â– â– iterm-â–
parity=(iterm%â– )â– â– -â–
tempâ– =tempâ– +parityâ– sin((@slopeyoutâ– tempout+temp)â– jterm)/(jterm^@poweryout)
elseif(@functypeyout=="cos odd alt")
jterm=â– â– iterm-â–
parity=(iterm%â– )â– â– -â–
tempâ– =tempâ– +parityâ– cos((@slopeyoutâ– tempout+temp)â– jterm)/(jterm^@poweryout)
elseif(@functypeyout=="sin all alt")
jterm=iterm
parity=(iterm%â– )â– â– -â–
tempâ– =tempâ– +parityâ– sin((@slopeyoutâ– tempout+temp)â– jterm)/(jterm^@poweryout)
elseif(@functypeyout=="cos all alt")
jterm=iterm
parity=(iterm%â– )â– â– -â–
tempâ– =tempâ– +parityâ– cos((@slopeyoutâ– tempout+temp)â– jterm)/(jterm^@poweryout)
elseif(@functypeyout=="sin general")
jterm=iterm
tempâ– =tempâ– +@funcyout(jterm)â– sin((@slopeyoutâ– tempout+temp)â– jterm)/(jterm^@poweryout)
elseif(@functypeyout=="cos general")
jterm=iterm
tempâ– =tempâ– +@funcyout(jterm)â– cos((@slopeyoutâ– tempout+temp)â– jterm)/(jterm^@poweryout)
endif
endwhile
ynew=y+@hyâ– tempâ–
x=xnew
y=ynew
z=x+flip(y)
endif
bailout:
|z|<@bailout default: title="Fourier Popcorn" heading text="Uses Cliff Pickover's Popcorn routine, substituting Fourier \ series for the sine and tangent functions." endheading ; ; general parameters ; heading caption="General parameters" endheading param control_type caption="control type" default=â– enum="basic" "advanced" endparam float param bailout caption="bailout value" default=â– â– â– â– endparam ; ; basic parameters ; heading caption="Fourier series parameters" visible=(@control_type=="basic") endheading complex param hall caption="step size" default=â– .â– visible=(@control_type=="basic") endparam param functypeall caption="function type" default=â– enum="sin odd pos" "cos odd pos" "sin all pos" "cos all pos" \ "sin odd alt" "cos odd alt" "sin all alt" "cos all alt" \ "sin general" "cos general" visible=(@control_type=="basic") endparam complex param powerall caption="power" default=â– visible=(@control_type=="basic") endparam int param ntermall caption="# terms" default=â– â– min=â– visible=(@control_type=="basic") endparam complex param slopeall caption="slope" default=â– visible=(@control_type=="basic") endparam func funcall caption="function" default=tan() visible=((@control_type=="basic")&&((@functypeall=="sin general")\ ||(@functypeall=="cos general"))) endfunc ; ; advanced parameters ; ; x inner parameters ; heading caption="x inner parameters" visible=(@control_type=="advanced") endheading complex param hx caption="step size" default=â– .â– visible=(@control_type=="advanced") endparam param vartypexin caption="inner variable type" default=â– enum="x" "y" "x+y" "y-x" "xâ– y" "y/x" visible=(@control_type=="advanced") endparam param functypexin caption="function type" default=â– enum="sin odd pos" "cos odd pos" "sin all pos" "cos all pos" \ "sin odd alt" "cos odd alt" "sin all alt" "cos all alt" \ "sin general" "cos general" visible=(@control_type=="advanced") endparam complex param powerxin caption="power" default=â– visible=(@control_type=="advanced") endparam int param ntermxin caption="# terms" default=â– â– min=â– visible=(@control_type=="advanced") endparam func funcxin caption="function" default=cos() visible=((@control_type=="advanced")&&((@functypexin=="sin general")\ ||(@functypexin=="cos general"))) endfunc ; ; x outer parameters ; heading caption="x outer parameters" visible=(@control_type=="advanced") endheading param vartypexout caption="outer variable type" default=â– enum="x" "y" "x+y" "y-x" "xâ– y" "y/x" visible=(@control_type=="advanced") endparam param functypexout caption="function type" default=â– enum="sin odd pos" "cos odd pos" "sin all pos" "cos all pos" \ "sin odd alt" "cos odd alt" "sin all alt" "cos all alt" \ "sin general" "cos general" visible=(@control_type=="advanced") endparam complex param powerxout caption="power" default=â– visible=(@control_type=="advanced") endparam int param ntermxout caption="# terms" default=â– â– min=â– visible=(@control_type=="advanced") endparam complex param slopexout caption="slope" default=â– visible=(@control_type=="advanced") endparam func funcxout caption="function" default=cos() visible=((@control_type=="advanced")&&((@functypexout=="sin general")\ ||(@functypexout=="cos general"))) endfunc ; ; y inner parameters ; heading caption="y inner parameters" visible=(@control_type=="advanced") endheading complex param hy caption="step size" default=â– .â– visible=(@control_type=="advanced") endparam param vartypeyin caption="inner variable type" default=â– enum="x" "y" "x+y" "y-x" "xâ– y" "y/x" visible=(@control_type=="advanced") endparam param functypeyin caption="function type" default=â– enum="sin odd pos" "cos odd pos" "sin all pos" "cos all pos" \ "sin odd alt" "cos odd alt" "sin all alt" "cos all alt" \ "sin general" "cos general" visible=(@control_type=="advanced") endparam complex param poweryin caption="power" default=â– visible=(@control_type=="advanced") endparam int param ntermyin caption="# terms" default=â– â– min=â– visible=(@control_type=="advanced") endparam func funcyin caption="function" default=sin() visible=((@control_type=="advanced")&&((@functypeyin=="sin general")\ ||(@functypeyin=="cos general"))) endfunc ; ; y outer parameters ; heading caption="y outer parameters" visible=(@control_type=="advanced") endheading param vartypeyout caption="outer variable type" default=â– enum="x" "y" "x+y" "y-x" "xâ– y" "y/x" visible=(@control_type=="advanced") endparam param functypeyout caption="function type" default=â– enum="sin odd pos" "cos odd pos" "sin all pos" "cos all pos" \ "sin odd alt" "cos odd alt" "sin all alt" "cos all alt" \ "sin general" "cos general" visible=(@control_type=="advanced") endparam complex param poweryout caption="power" default=â– visible=(@control_type=="advanced") endparam int param ntermyout caption="# terms" default=â– â– min=â– visible=(@control_type=="advanced") endparam complex param slopeyout caption="slope" default=â– visible=(@control_type=="advanced") endparam func funcyout caption="function" default=sin() visible=((@control_type=="advanced")&&((@functypeyout=="sin general")\ ||(@functypeyout=="cos general"))) endfunc } mt-bof-newton-I-m { ; Mark Townsend, â– â– Mar â– â– â– â– ; ; Modified Newton's method for ; (z-â– )â– (z+c)â– (z^â– +â– ), from "The Beauty of ; Fractals". ; init: z = â– c = #pixel float h = â– .â– â– â– â– fz = zh = oldz = â– loop: oldz = z zh = z + h fz = (z - â– ) â– (z + c) â– (z^â– + â– ) fzd = â– / h â– ((zh - â– ) â– (zh + c) â– (zh^â– + â– ) - fz) z = z - fz /(fzd + flip(@s)) bailout: |z - oldz| > @epsilon
default:
title = "Bof Newton I"
magn = â– .â– â–
maxiter = â– â– â– â–
periodicity = â–
param s
caption = "Parameter (s)"
default = â– .â–
hint = "The suggested range is â– <s<â– ." endparam="" param="" epsilon="" caption="Epsilon" default="â– e-â– " hint="This is the bailout value." switch:="" type="mt-bof-newton-I-j" s="s" c="#pixel" bailout="bailout" }<="" pre=""></s<â– .">
